An Understanding of Basic Scheduling Process and Terms

Introduction

For any project irrespective of size, whether small or big, the first and foremost strategy that should be ignited is how to manage the delivery of the project. Hence, the first action that should come to the mind of one who is going to manage the project is to divide the overall scope at the activities level, i.e., manageable level. Effective management of a project requires concentrating the work at the activities level so that the overall execution of the project can be achieved at the desired level.

The duration required for execution and completion of each activity shall be assessed based on the productivity rate per unit, that could be achieved taking into consideration the many factors. This is an important factor that requires careful attention in developing a realistic schedule. The activities identified for completion are to be placed in a sequential manner called logical sequence, in which the relationship between each activity plays a key role. This relationship among activities can be determined based on the combination of technical requirements and the expected completion of a certain facility at some point in time, prior to completing the overall facility.

Thus, the overall scope of each activity, activity productivity rate per unit, activity duration, the relationship between activities, and finally placing those activities in a logical sequence are the important steps to making a successful program.

Scheduling on Activity-on-Node (AoN) by Critical Path Method (CPM)

The computer-generated Critical Path Method (CPM) schedule refers to numerous terminologies for program users. However, those terms are often complex to understand for managers who did not familiar with such terms. A clear understanding of those basic terms will assist managers to understand how to make an effective program, read programs, and monitor actual progress in line with the program.

In the modern world, CPM is predominantly used for developing a program and, specifically, for major and complex projects dealing with tens of thousands of activities. Thus, any attempt to understand the progress status of a project on the CPM program, without being familiar with CPM scheduling techniques, would be a waste of time.

A more understanding of scheduling terms requires understanding the calculation of values of those basic terms such as the early and late start, early and late finish and floats, etc. These understandings would make managers more knowledgeable about the importance of the sequence of activities and its interlinking with activity duration, the start and completion date of activities, the project completion date, and float values.

Any changes to the planned sequence of activities affect the start and completion date of the planned activities and the float values.

Definition of Scheduling Terms

The definitions of these basic terms are listed below:

1. Activity-on-Node (AON) diagram – It is a logic diagram that graphically illustrates the logical flow of the work sequence planned.
2. Early Start (ES) – The earliest Date the activity can start.
3. Early Finish (EF) – Earliest Date the activity can finish.
4. Late Start (LS) – Latest date the activity can start
5. Latest Finish (LF) – Latest date the activity can finish.
6. Critical activity – any activity that contains no float is a critical activity.
7. Critical Path – A critical path is the chain of critical activities from the project’s inception to completion. The sum of the activity duration in the chain of critical activity is the project’s duration. Therefore, any delay in the critical activity will affect the project’s completion.
8. Lag – A planned/necessary wait time between activities.
9. Lead – a time that a successor can start before the completion of the predecessor activity. This is ideally being used to manage time effectively (i.e., in lieu of FF, SS)
10. Total float – the maximum number of days an activity can be delayed without delaying the project completion date.
11. Free float – the maximum number of days an activity can be delayed before delaying the successor activity.
12. Predecessor activity – before the activity
13. Successor activity -= after activity

The predecessor and successor activity can be understood when there are two activities such as “A” and “B”. The relationship between “A” and “B” is Finish to Start, i.e. to start Activity “B”, Activity “A” should be 100% completed. In such a relationship, Activity “A” is a predecessor to Activity “B”, whilst Activity “B” is a successor Activity to “A”

The values of early start/finish, late start/finish, total float, and free float are calculated by Forward Pass and Backward Pass.

Forward Pass

The forward pass is to run through activities logically placed from inception to completion. There are two activities with 5 days duration each, as follows:

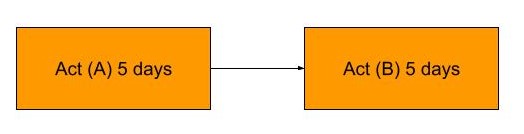
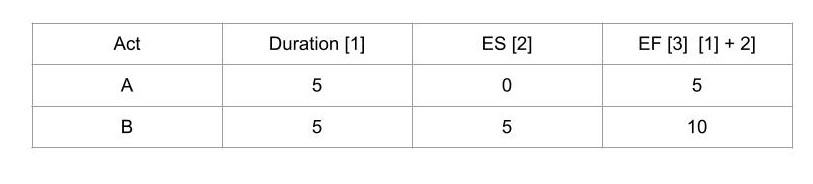
The ES of Activity A begins with “zero” and accordingly the values of EF of Activity A and ES and EF of successor activities are calculated with the formulas as follows:

ES = Maximum (or highest) EF value from an immediate predecessor.

EF = ES + Duration

The ES and EF calculations are as follows:                                     

However, if there are four (4) activities, A – D, logically placed with FS relationship as follows:

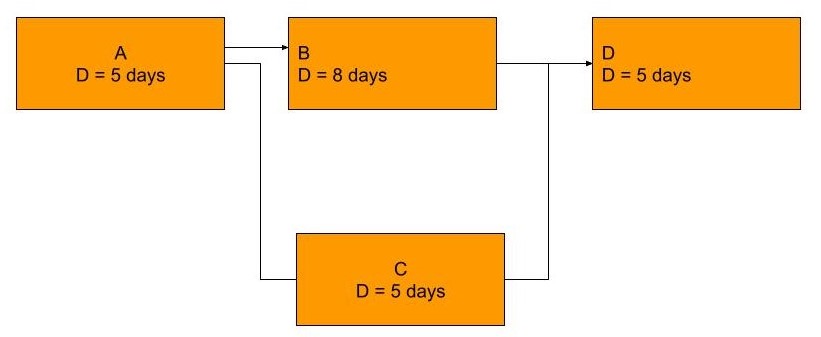
The ES and EF calculations are as follows:

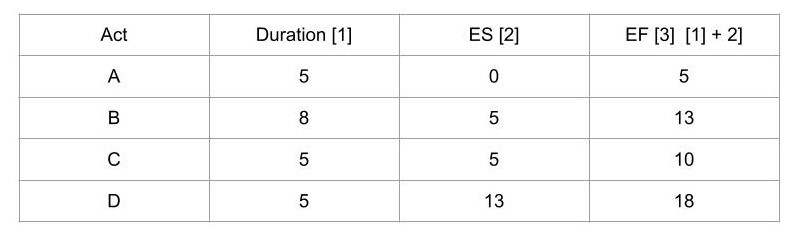
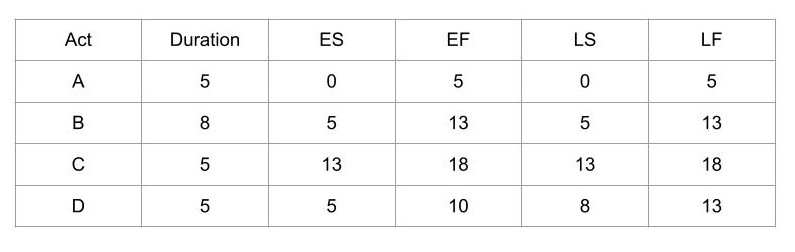
In the above context, the ES calculation for Activity D is slightly different from the usual method of carrying the EF value of the preceding activity. If there were more than one preceding activity for an activity, the ES calculation for such (successor) activity shall take the maximum or higher value of EF which a predecessor has among all predecessors. As such, since Activity D has two predecessors, the ES value of Activity D shall be 13, which is the maximum/higher EF value among Activity B and C.

Backward Pass

The Backward Pass is to run through activities logically placed from completion to inception. There are two activities with 5 days duration on each.

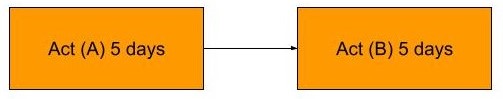
The LF of Activity B is the same value of the EF of Activity B. As such LF of Activity B is 10 using the following formula, and details refer to below table.

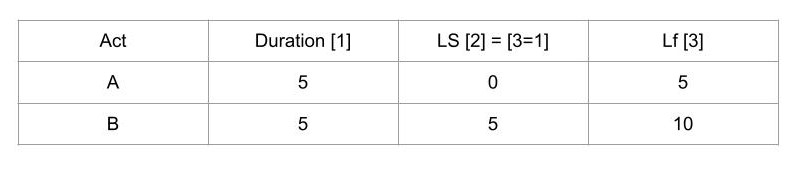
LF = Minimum (or lowest) LS value from immediate successor(s); LS = LF – Duration

The LS and LF calculations are as follows:

However, if there are four (4) activities, A – D, logically placed with FS relationship as follows.

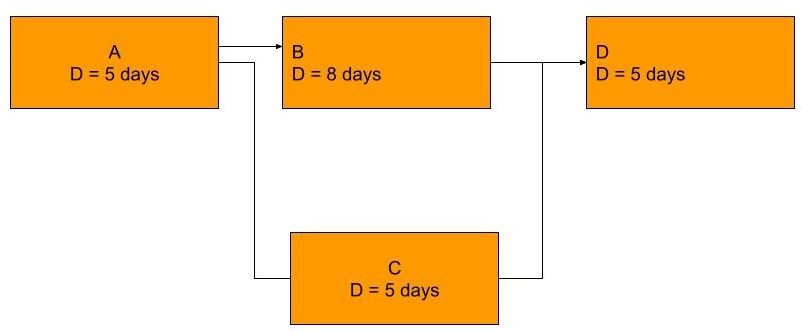
The LS and LF calculations are as follows:

The LF calculation for Activity A is slightly different from the usual method of carrying the LS of the successor activity. If there were more than one successor activity for an activity, the lowest start date of the successor activity will be carried as the LF of the predecessor activity. As such, since Activity A has two successors, and Activity B has the lowest value of LS, the same value shall be carried out to establish the LF value of Activity A.

The compilation of the values derived through Forward and Backward Passes is below:

Float

Floats are of two types, 1) total float, and 2) free float.

The total float represents the difference between the latest start date of the activity and the earliest start date of the activity. Similarly, the difference between the Late finish date of the activity and the earliest finish date of the activity, i.e., LS-ES and LF-EF. If an activity has a total float of 10 days (i.e., LS-ES and LF-EF), it means the activity can be delayed to a maximum of 10 days before the project completion is delayed.

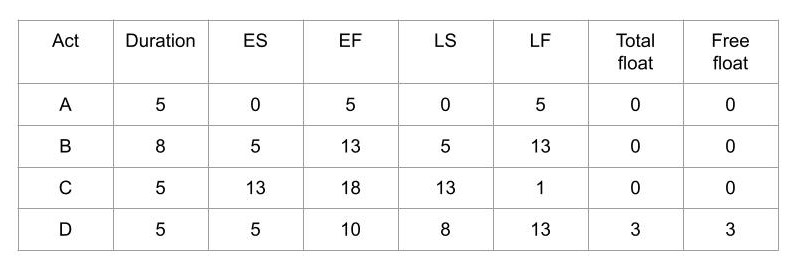
Conversely, the free float is the difference between the early finish date of the predecessor activity and the early start date of the successor activity. In the above example, activity D has three days of free float, i.e., activity D can be delayed up to a maximum of three days without delaying the successor activity C.

Critical Activity and Path

The activities that have no float are critical to the program’s completion. Zero floats give no cushion to absorb any delay and thus identifying zero floats’ activities is crucial for the successful completion of the activity. The string of critical activities creates a path called Critical Path. The sum of the duration of critical path activities establishes the project duration. In the given example, the critical activities are A, B, and C, and as such these activities’ duration establishes the project duration of 18 (5+8+5).

Conclusion

This article demonstrates the basics of developing a schedule including the basic scheduling terms, together with the calculation of the value of scheduling terms using the Activity on Node method (AoN). The article necessitates an understanding of the scheduling basis terms for the successful planning, execution, and monitoring of works, etc.